Plotting the root locus
Adding a notch filter
Finding the gain from the root locus
Plotting closed-loop response

From the main problem, the dynamic equations in transfer function form are the following:

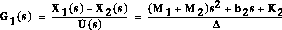
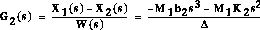
For the original problem setup and the derivation of the above equations and schematic, please refer to the bus modeling page.

If you are interested in running an animation of this example based on the control techniques used in the root locus tutorial please go to the bus suspension animation page after completing this tutorial.

We want to design a feedback controller so that when the road disturbance (W) is simulated by a unit step input, the output (X1-X2) has a settling time less than 5 seconds and an overshoot less than 5%. For example, when the bus runs onto a 10 cm high step, the bus body will oscillate within a range of +/- 5 mm and will stop oscillating within 5 seconds.

The system model can be represented in Matlab by creating a new m-file and entering the following commands (refer to main problem for the details of getting those commands).

m1=2500;
m2=320;
k1 = 80000;
k2 = 500000;
b1 = 350;
b2 = 15020;

nump=[(m1+m2) b2 k2]
denp=[(m1*m2) (m1*(b1+b2))+(m2*b1) (m1*(k1+k2))+(m2*k1)+(b1*b2) (b1*k2)+(b2*k1) k1*k2]

num1=[-(m1*b2) -(m1*k2) 0 0]
den1=[(m1*m2) (m1*(b1+b2))+(m2*b1) (m1*(k1+k2))+(m2*k1)+(b1*b2) (b1*k2)+(b2*k1) k1*k2]

numf=num1;
denf=nump;

First let's see what the open loop poles of the system are:

R=roots(denp)

R =
  -23.9758 +35.1869i
  -23.9758 -35.1869i
  -0.1098 + 5.2504i
  -0.1098 - 5.2504i
      

Plotting the root locus

The main idea of root locus design is to estimate the closed-loop response from the open-loop root locus plot. By adding zeros and/or poles to the original system (adding a compensator), the root locus and thus the closed-loop response will be modified. Let's first view the root locus for the plant. In your m-file, add the following command and then run the file, you should get the root locus plot below:

rlocus(nump,denp)
z=-log(0.05)/sqrt(pi^2+(log(0.05)^2))
sgrid(z,0)

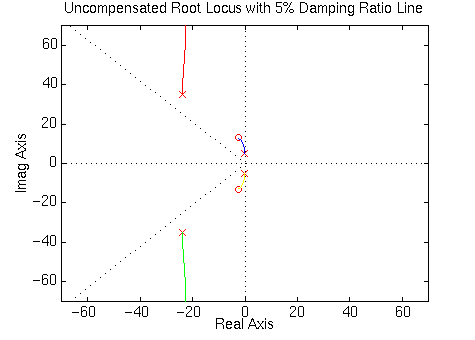
Note from the specification, we required the overshoot, %OS, to be less than 5% and damping ratio, zeta, can be find from approximation damping ratio equation, z = -log(%OS/100)/sqrt(pi^2+[log(%OS/100)^2]). The commandsgrid is used to overlay desired percent overshoot line on the close-up root locus, you can find more information from commands list.

From the plot above, we see that there are two pair of the poles and zeros that are very close together. These pair of poles and zeros are almost on the imaginary axis, they might make the bus system marginally stable, which might cause a problem. We have to make all of the poles and zeros move into the left-half plane as far as possible to avoid an unstable system. We have to put two zeros very close to the two poles on the imaginary axis of uncompensated system for pole-and-zero cancellation. Moreover, we put another two poles further on the real axis to get fast response.

Adding a notch filter

We will probably need two zeros near the two poles on the complex axis to draw the root locus, leaving those poles to the compensator zeros instead of to the plant zeros on the imaginary axis. We'll also need two poles placed far to the left to pull the locus to the left. It seems that a notch filter (2-lead controller) will probably do the job. Let's try putting the zeros at 30 and 60 and the poles at 3+/-3.5i. In your m-file add the following lines of code:

z1=3+3.5i;
z2=3-3.5i;
p1=30;
p2=60;
numc=conv([1 z1],[1 z2]);
denc=conv([1 p1],[1 p2]);
rlocus(conv(nump,numc),conv(denp,denc))

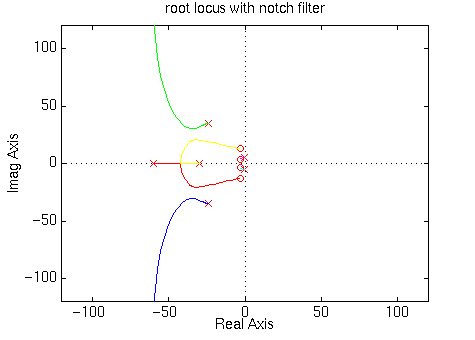
rlocus(conv(nump,numc),conv(denp,denc))
axis([-40 10 -30 30])
z=-log(0.05)/sqrt(pi^2+(log(0.05)^2))
sgrid(z,0)

:

Finding the gain from the root locus

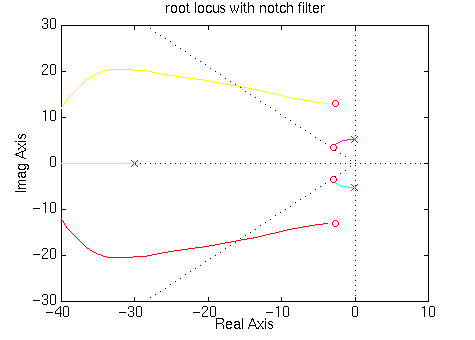
Now that we have moved the root locus across the 5% damping ratio line, we can choose a gain that will satisfy the design requirements. Recall that we want the settling time and the overshoot to be as small as possible. Generally, to get a small overshoot and a fast response, we need to select a gain corresponding to a point on the root locus near the real axis and far from the complex axis or the point that the root locus crosses the desired damping ratio line. But in this case, we need the cancellation of poles and zeros near the imaginary axis, so we need to select a gain corresponding to a point on the root locus near zeros and percent overshoot line. There is a method to do this with the rlocfind command in matlab. Enter the following command into the Matlab command window:

[k,poles]=rlocfind(conv(nump,numc),conv(denp,denc))

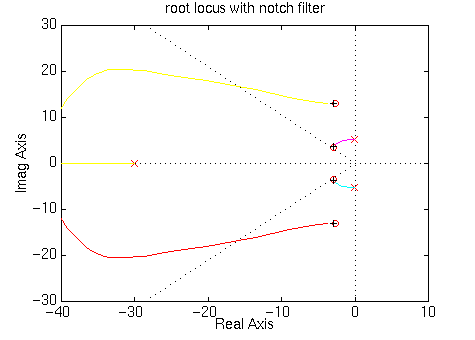
selected_point =

  -2.9428 -13.0435i


K =

   1.0678e+08


poles =

   1.0e+02 *

  -0.6322 + 6.1536i
  -0.6322 - 6.1536i
  -0.0294 + 0.1306i
  -0.0294 - 0.1306i
  -0.0292 + 0.0367i
  -0.0292 - 0.0367i

numc=k*numc;

and the closed-loop transfer function can be derived as following:

To obtain the closed-loop transfer function from W to X1-X2, add the following to your m-file:

numa=conv(conv(numf,nump),denc);
dena=conv(denf,polyadd(conv(denp,denc),conv(nump,numc)));

Plotting the closed-loop response

Let's see what the closed-loop step response looks like with this compensator. Keep in mind that we are going to use a 0.1 m high step as the disturbance. To simulate this, simply multiply numa by 0.1. Add the following commands into the m-file and put % marks in front of all rlocus and rlocfind commands.

step(0.1*numa,dena)
title('closed-loop response to 0.1m high step w/ notch filter')

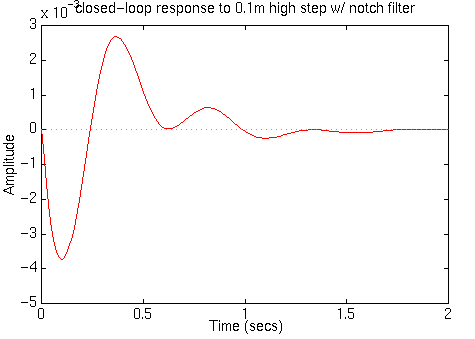
Note: A design problem does not necessarily have an unique answer. Using the root locus method (or any other method) may result in many different compensators. For practice, you may want to go back to the original open-loop root locus and try to find other good ways to add zeros and poles to get a better response.

If you are interested in running an animation of the bus suspension example based on the control techniques used in this tutorial please go to the Bus Suspension Animation Page.

Root Locus Examples

Cruise Control | Motor Speed | Motor Position | Bus Suspension | Inverted Pendulum | Pitch Controller | Ball and Beam

Bus Suspension Examples

Modeling | PID | Root Locus | Frequency Response | State Space | Digital | Animation

Tutorials

Basics | Modeling | PID | Root Locus | Frequency Response | State Space | Examples